
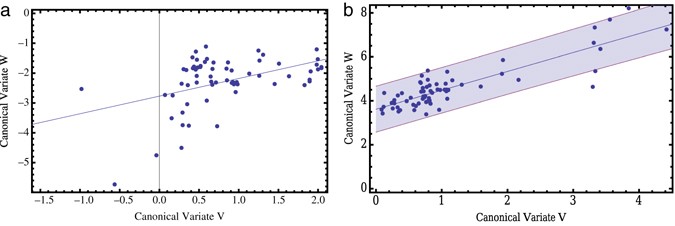
Drawing the many axes is actually needless because the space has the number of non-redundant dimensions equal to the number of non-collinear variables. Variable points are connected with the origin and form vectors, arrows, spanning the subject space so here we are ( see also). In a subject space, if variables have been centered, the cosine of the angle between their vectors is Pearson correlation between them, and the vectors' lengths squared are their variances. On the pictures below the variables displayed are centered (no need for a constant arises). Variables $X_1$ and $X_2$ positively correlate: they have acute angle between them. Principal components $P_1$ and $P_2$ lie in the same space "plane X" spanned by the two variables.
The components are variables too, only mutually orthogonal (uncorrelated).

The direction of $P_1$ is such as to maximize the sum of the two squared loadings of this component and $P_2$, the remaining component, goes orthogonally to $P_1$ in plane X. The squared lengths of all the four vectors are their variances (the variance of a component is the aforementioned sum of its squared loadings). Component loadings are the coordinates of variables onto the components - $a$'s shown on the left pic.


 0 kommentar(er)
0 kommentar(er)
